How To Draw Hyperbola
How To Draw Hyperbola - This ratio is called the eccentricity, and for a hyperbola it is always greater than 1. Graph the hyperbola given by the equation y2 64 − x2 36 = 1 y 2 64 − x 2 36 = 1. Graphing a hyperbola centered at (0, 0) given an equation in standard form. Yang kuang and elleyne kase. Draw diagonal lines through the center and the corners of the rectangle that extend beyond the rectangle. Any branch of a hyperbola can also be defined as a curve where the distances of any point from: Sometimes the equation for a hyperbola needs to be first placed in standard form before we graph it. (3x − 2)2 − 3y2 = 1 ( 3 x − 2) 2 − 3 y 2 = 1. A fixed straight line (the directrix) are always in the same ratio.; 6.4 hyperbolic functions (ema4p) functions of the form \ (y=\dfrac {1} {x}\) (ema4q) functions of the general form \ (y=\dfrac {a} {x}+q\) are called hyperbolic functions. Draw diagonal lines through the center and the corners of the rectangle that extend beyond the rectangle. Sometimes the equation for a hyperbola needs to be first placed in standard form before we graph it. A fixed straight line (the directrix) are always in the same ratio.; Web use these points to draw a rectangle that will help guide the. Explore book buy on amazon. Determine if it is horizontal or vertical. Label the foci and asymptotes, and draw a smooth curve to form the hyperbola, as shown in figure 8. Draw diagonal lines through the center and the corners of the rectangle that extend beyond the rectangle. 11k views 8 years ago. Explore book buy on amazon. In this maths lesson we learn how to draw a hyperbola. Determine if it is horizontal or vertical. Web sketch and extend the diagonals of the central rectangle to show the asymptotes. Graph the hyperbola given by the equation y2 64 − x2 36 = 1 y 2 64 − x 2 36 = 1. Web sketch and extend the diagonals of the central rectangle to show the asymptotes. Graphing a hyperbola centered at (0, 0) given an equation in standard form. (y−k)2 b2 − (x−h)2 a2 = 1 ( y − k) 2 b 2 − ( x − h) 2 a 2 = 1. Explore book buy on amazon. Label the foci and. Drawing the asymptotes and the vertices will get you closest to a graph. Yang kuang and elleyne kase. Web sal introduces the standard equation for hyperbolas, and how it can be used in order to determine the direction of the hyperbola and its vertices. Draw diagonal lines through the center and the corners of the rectangle that extend beyond the. To clarify how this method works, the string to the pen represents. Graphing a hyperbola centered at \((0,0)\) given an equation in standard form (h,k) ( h, k) (h,k) ( h, k) opens. A fixed point (the focus), and; The two lines that the hyperbolas come closer and closer to touching. When graphing a hyperbola, you can think of it as a mix of two parabolas — each one a perfect mirror image of the other, and each opening away. (h,k) ( h, k) (h,k) ( h, k) opens. Web sal introduces the standard equation for hyperbolas, and how it can be used in order to determine the direction of the. In this video, i will show you how to draw a. Web to graph a hyperbola, mark points \(a\) units left and right from the center and points \(b\) units up and down from the center. Graph the hyperbola given by the equation y2 64 − x2 36 = 1 y 2 64 − x 2 36 = 1. Graph. Web in this video, i have explained how to draw an hyperbola by eccentricity method, also how to draw a tangent and a normal to the hyperbola curve. A fixed point (the focus), and; When graphing a hyperbola, you can think of it as a mix of two parabolas — each one a perfect mirror image of the other, and. In this video, i will show you how to draw a. \ (y=h\left (x\right)=\dfrac {1} {x}\) (3x − 2)2 − 3y2 = 1 ( 3 x − 2) 2 − 3 y 2 = 1. Web use these points to draw a rectangle that will help guide the shape of your hyperbola. Use the a value to find the two. Graph the hyperbola given by the equation y2 64 − x2 36 = 1 y 2 64 − x 2 36 = 1. A fixed point (the focus), and; (h,k) ( h, k) (h,k) ( h, k) opens. 6.4 hyperbolic functions (ema4p) functions of the form \ (y=\dfrac {1} {x}\) (ema4q) functions of the general form \ (y=\dfrac {a} {x}+q\) are called hyperbolic functions. Going left and right 3 gives you a width of 6. A 2 + b 2 = c 2. Explore book buy on amazon. (y−k)2 b2 − (x−h)2 a2 = 1 ( y − k) 2 b 2 − ( x − h) 2 a 2 = 1. The central rectangle and asymptotes provide the framework needed to sketch an accurate graph of the hyperbola. First, we know this is horizontal since the x is positive. 27k views 3 years ago technical & engineering drawing. The two lines that the hyperbolas come closer and closer to touching. Use the a value to find the two vertices. Web sal introduces the standard equation for hyperbolas, and how it can be used in order to determine the direction of the hyperbola and its vertices. Web here is a table giving each form as well as the information we can get from each one. Web in this video, i have explained how to draw an hyperbola by eccentricity method, also how to draw a tangent and a normal to the hyperbola curve.
HOW TO DRAW THE RECTANGULAR HYPERBOLA IN ENGINEERING DRAWING YouTube

HOW TO DRAW HYPERBOLA IN ENGINEERING DRAWING YouTube

How to sketch a hyperbola by hand YouTube

How to draw a Hyperbola by Arcs of Circle Method YouTube

How to draw Hyperbola by GeoGebra classic6 part2. YouTube

How to Draw a HYPERBOLA in Engineering Drawing What is a Hyperbola

Easy Steps to Draw A Hyperbola using Focus Directrix Method Engg
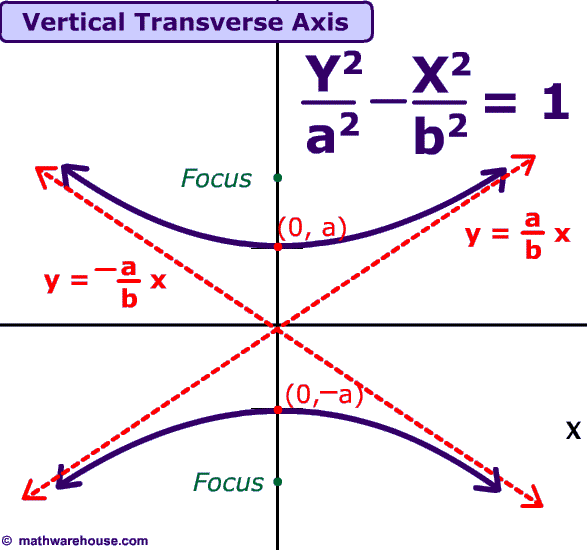
Formula and graph of a hyperbola. How to graph a hyperbola based on its
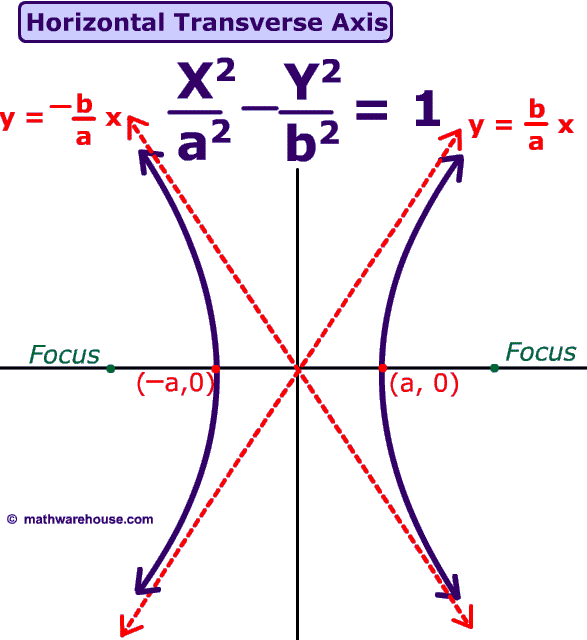
Formula and graph of a hyperbola. How to graph a hyperbola based on its

hyperbola sketch YouTube
Any Branch Of A Hyperbola Can Also Be Defined As A Curve Where The Distances Of Any Point From:
Web Sketch And Extend The Diagonals Of The Central Rectangle To Show The Asymptotes.
This Ratio Is Called The Eccentricity, And For A Hyperbola It Is Always Greater Than 1.
The Lines Through The Corners Of This Rectangle Are The Asymptotes.
Related Post: